Stochastic processes have a tremendous importance in fields such as economy, politics, biology, marketting, chemistry and, of course, mathematics. It's applications range from weather forecasts, electoral polls, epidemic dissemination, chemical kinetics, stock exchange fluctuations, etc. Brownian motion is on of the simplest stochastic processes one may consider.

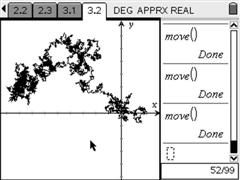
The principles involved in brownian motion are quite simple: consider a particle in the surface of a lake. No matter how still is the water and how small the wind, there are always miniscule fluctuations that will cause the particle to move in a random direction. Being a tiny ondulation, a very gentle brise or even intermolecular forces.

After some time the completely random motion of the particle will display some very interesting features: some regions seem to atract the particle, while others seem to be avoided. Different simulations will differ but some properties will remain. This simulator allows one to get acquainted to stochastic processes and how they sometimes contradict our intuition of what's random.

Download the Brownian motion simulator in:

• English
• Portuguese